Tensor Networks Initiative

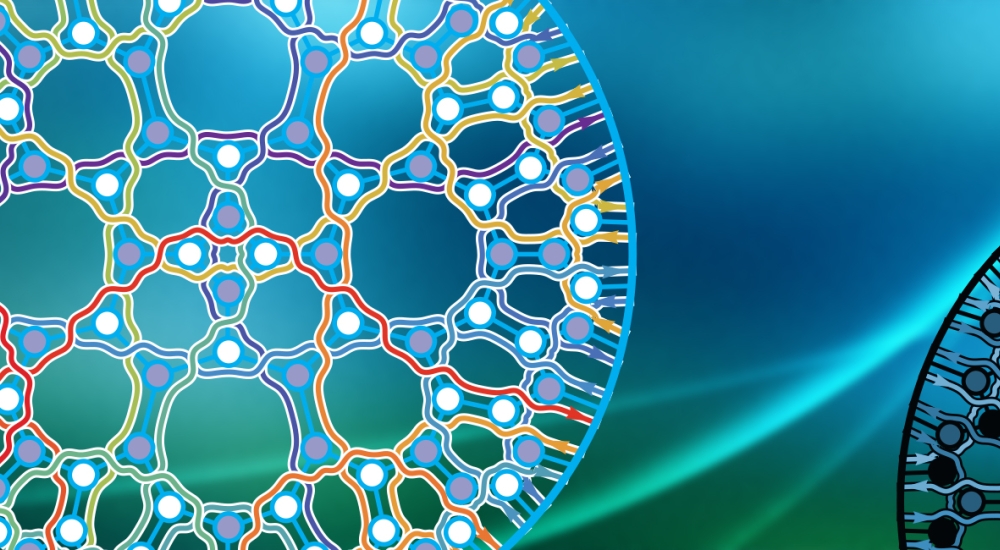
From entangled quantum matter to emergent space time

Tensor networks have in recent years emerged as a powerful tool to describe strongly entangled quantum many-body systems. Applications range from the study of collective phenomena in condensed matter to providing a discrete realization of the holographic principle in quantum gravity.

Tensor networks – such as the matrix product state (MPS), the multi-scale entanglement renormalization ansatz (MERA), and the projected entangled-pair states (PEPS) – were originally proposed as novel numerical approaches to study strongly entangled quantum many-body systems, including quantum criticality and topological order. However, the range of applicability of the tensor network formalism has quickly extended well beyond the computational domain.

Tensor networks are currently also investigated as a natural framework to classify exotic phases of quantum matter, as the basis for new non-perturbative formulations of the renormalization group and interacting quantum field theories, as a lattice realization of the AdS/CFT correspondence in quantum gravity, and in machine learning.

Topics that the tensor network initiative at Perimeter Institute explores include:

Strongly entangled quantum matter: characterization of exotic phases and critical phenomena
Novel non-perturbative approaches to quantum field theories, including their dynamics
Tensor network realization of the AdS/CFT correspondence
New theoretical and computational frameworks for quantum gravity
Machine learning

Juan Maldacena and Guifre Vidal talking at Perimeter Institute — Juan Maldacena, who first proposed the AdS/CFT correspondence in 1997, talks to Perimeter Faculty member Guifre Vidal about tensor networks as a realization of the AdS/CFT correspondence at Mathematica Summer School 2015.

Researchers:

Faculty:

Research Staff:

Erik Schnetter

Postdocs:

Jacob Bridgeman
Martin Ganahl
Stefan Kühn
Adam Lewis
Ashley Milsted

Students:

Qi Hu
Sebastian Mizera
Adrian Franco Rubio
Ruoshui Wang
Yijian Zou

Distinguished Visiting
Research Chairs:

Ignacio Cirac
Frank Verstraete
Xiao-Gang Wen
Steve White

Visiting Fellows:

Philippe Corboz
Glen Evenbly
Jutho Haegeman
Brian Swingle

Advisory committee:

Workshops and Conferences supported by or related to the Tensor Networks Initiative:

Papers

Glen Evenbly, Guifre Vidal, “Tensor network renormalization yields the multi-scale entanglement renormalization ansatz”, arXiv:1502.05385.
E.M. Stoudenmire, David J. Clarke, Roger S. K. Mong, and Jason Alicea, "Assembling Fibonacci Anyons From a Z3 Parafermion Lattice Model", arXiv:1501.05305.
Glen Evenbly, Guifre Vidal, “Tensor Network Renormalization”, arXiv:1412.0732.
Shuo Yang, Thorsten B. Wahl, Hong-Hao Tu, Norbert Schuch, J. Ignacio Cirac, "Chiral projected entangled-pair state with topological order", Phys. Rev. Lett. 114, 106803 (2015), arXiv:1411.6618.
Ho N. Phien, Ian P. McCulloch, Guifre Vidal, “Faster convergence of imaginary time evolution tensor network algorithms by recycling the environment”, arXiv:1411.0391.
E.M. Stoudenmire, Peter Gustainis, Ravi Johal, Stefan Wessel, and Roger G. Melko, "Corner Contributions to the Entanglement Entropy of Strongly-Interacting O(2) Quantum Critical Systems in 2+1 Dimensions," Phys. Rev. B, 90: 235106, arXiv: 1409.6327.
Bianca Dittrich, Sebastian Mizera, Sebastian Steinhaus, "Decorated tensor network renormalization for lattice gauge theories and spin foam models," arXiv: 1409.2407.
Lucas O. Wagner, Thomas E. Baker, E.M. Stoudenmire, Kieron Burke, and Steven R. White, "Kohn-Sham Calculations with the Exact Functional", Phys. Rev. B, 90: 045109 (2014), arXiv.org: 1405.0864.
Ann Kallin, E.M. Stoudenmire, Paul Fendley, Rajiv R.P. Singh, Roger G. Melko, "Corner contribution to the entanglement entropy of an O(3) quantum critical point in 2+1 dimensions", J. Stat. Mech. P06009 (2013), arXiv: 1401.3504.
B. Bauer, L. Cincio, B. P. Keller, M. Dolfi, G. Vidal, S. Trebst, A. W. W. Ludwig, "Chiral spin liquid and emergent anyons in a Kagome lattice Mott insulator," (accepted in Nature Communications), arXiv: 1401.3017.
Bianca Dittrich, Mercedes Martin-Benito, Sebastian Steinhaus, "Quantum group spin nets: refinement limit and relation to spin foams," Phys. Rev. D 90, 024058 (2014), arXiv: 1312.0905.
Glen Evenbly, Guifre Vidal, "Algorithms for entanglement renormalization: boundaries, impurities and interfaces," J Stat Phys, DOI 10.1007/s10955-014-0983-1, arXiv: 1312.0303.
Bianca Dittrich, Sebastian Steinhaus, "Time evolution as refining, coarse graining and entangling," arXiv: 1311.7565.
Bianca Dittrich, Wojciech Kaminski, "Topological lattice field theories from intertwiner dynamics," arXiv: 1311.1798.
Glen Evenbly, Guifre Vidal, "Scaling of entanglement entropy in the (branching) multi-scale entanglement renormalization ansatz," Phys. Rev. B 89, 235113 (2014), arXiv: 1310.8372.
Bianca Dittrich, Mercedes Martín-Benito, Erik Schnetter, "Coarse graining of spin net models: dynamics of intertwiners," New J. Phys. 15 (2013) 103004, arXiv: 1306.2987.
Glen Evenbly, Guifre Vidal, "A class of highly entangled many-body states that can be efficiently simulated," Phys. Rev. Lett. 112, 240502 (2014), arXiv: 1210.1895.
Glen Evenbly and Guifre Vidal, "A real space decoupling transformation for quantum many-body systems," Phys. Rev. Lett. 112, 220502 (2014), arXiv: 1205.0639.

Tensor Networks Initiative

From entangled quantum matter to emergent space time

Researchers:

Advisory committee:

Lectures and talks