Quantum technologies are widely viewed as the next summit in computing – but there are many ways to the top. Researchers around the world are pursuing different approaches to develop robust and scalable quantum computing schemes, harnessing everything from optical lasers to quantum entanglement.

Yet one scheme has long seemed too exotic to be considered practical. It was deemed too theoretical, too far from being testable. But, thanks to a new paper by a Canada-based collaboration, it might not remain off the beaten path for long.

In the paper “Experimental Identification of Non-Abelian Topological Orders on a Quantum Simulator,” published in Physical Review Letters in February, theorists and experimentalists have carved out a middle ground between exotic theory and achievable experiment.

The collaboration has shown how a small, circuit-based quantum computer can be used to simulate something called topological quantum computing.

By doing so, they’ve taken the first step in a research program they hope could lead to a fault-tolerant quantum computer that derives its power and stability not from the infinitesimal pieces that make up the quantum system, but from how the entire system behaves.

Former Perimeter postdoctoral researcher Yidun Wan.[/caption]

“I think it offers a new route to realize quantum computation,” said Yidun Wan, who launched the research project as a Perimeter postdoctoral fellow in 2016, with fellow PI postdoc Ling-Yan Hung. Both are now professors at Fudan University in Shanghai.

“People either thought of quantum circuit models or topological quantum computation,” said Wan. “This is kind of a way in between. We utilize the advantages of both schemes.”

In theory, topological quantum computation (or TQC) is hugely promising. Topological matter earns its name because its behaviour is dictated by its shape: if you take two pieces of topological matter, shape one into a sphere and the other into a doughnut, and then use those different versions to process information, the systems that began as identical will deliver two entirely different results.

Because the computation is dictated by the collective entity, topological systems are predicted to be extremely robust. This is very different from other forms of quantum computing, which rely on manipulating single quantum bits, or qubits, and are extremely sensitive to perturbations from outside effects, making them vulnerable to glitches or “local errors.”

In practice, however, TQC is a long way from being realized; scientists haven’t yet settled what physical system they would use in order to try to create it.

So, instead of waiting to find the perfect physical system to experimentally demonstrate topological computation, Wan proposed using current technology – a circuit-based quantum computer – to simulate a topological quantum computer. The compromise was demonstrated experimentally by researchers at the Institute for Quantum Computing at the University of Waterloo, and the results have been hailed as something of a watershed moment. One of the paper’s referees described the results as “a milestone of the physical realization of TQC.”

The in-between route doesn’t provide all the power of true topological quantum computation, but it does give a glimpse of what could be possible.

The ingredients for quantum computation

The basic unit of classical computation is the bit. Information is stored as either a 1 or a 0, which works well but limits the amount of data that can be processed.

The quantum analog to your desktop computer is circuit-based quantum computing. Here, the basic unit is a qubit (short for quantum bit), which is usually a specific ion or electron. Because of its analogy to classical computing, circuit-based quantum computing is the traditional starting point for people entering the field. The main drawback of this approach is that it is open to local errors – from magnetic fields and other external perturbations. To counteract this, the system includes quantum error correction measures. This makes the computations more accurate, but it means the vast majority of the qubits are dedicated to correcting errors, rather than actual computation.

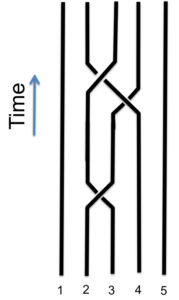
An example of how Fibonacci anyon pathways can braid through time.[/caption]

Topological quantum computation takes an entirely different approach. Rather than using specific particles as its qubits, TQC starts with anyons, an exotic kind of quasiparticle that is neither a fermion (electrons, protons, etc.), nor a boson (photons, gluons, etc.). These anyons are then moved in a particular way so that the tracks they leave behind end up braiding as they flow through time [see diagram, right].

Just like when braiding hair, TQC will only hold together if the anyons are braided one crossing at a time, and only in certain directions. (If an anyon crossed left and then right, without looping around, it could simply be pulled apart.) That turns each crossing of the anyon paths into a gate, where computation occurs. Because the computation is performed by the behaviour of the system, it is largely impervious to local errors. It is, in effect, fault tolerant.

There are a number of distinct quasiparticle types. When TQC was first proposed, in 1997, the idea harnessed a specific kind of quasiparticle called a non-Abelian anyon (which effectively means that changing the order of the anyons’ movements changes their final tracks). Of particular interest was something called a Fibonacci anyon. If that name sounds familiar, it’s with good reason. As the anyons interact and fuse, the dimensions in the system increase according to the Fibonacci sequence.

First, it helps to understand that the theory of anyons is placed in something called Hilbert space. This theoretical space of infinite dimensions arises often in physics and mathematics.

One anyon forms a one-dimensional Hilbert space, two anyons form a two-dimensional Hilbert space, and three anyons form a three-dimensional space. Then, things get interesting: four anyons form a five-dimensional Hilbert space, six form a 13-dimensional one, and so on. While that makes Fibonacci anyons sound incredibly complex, it is actually the simplest candidate that we know of for topological quantum computing.

In 2014, researchers at Cornell University and Microsoft’s Station Q theoretically showed that, as Fibonacci anyons move around each other and create braids and knots of tracks, they in effect become qubits. Three or four quasiparticles correspond to a single qubit; where the paths cross corresponds to a computing gate.

Proving that in experiment is incredibly challenging, though, so theoretical physicist Yidun Wan came up with a different approach. Instead of waiting until we discover a physical system in which Fibonacci anyons can be realized – a process that could take years – why not use today’s capabilities in circuit-based quantum computing to mimic TQC?

And he knew exactly who he’d ask to test the idea. The Institute for Quantum Computing sits just up the road from Perimeter, and experimentalists there have successfully created 12-qubit circuit systems that can be fully controlled. Wan figured that a three-qubit proof-of-concept experiment would be possible. He pitched the idea at an IQC seminar. The experimentalists bought in. The game was on.

Using the tools at hand

IQC postdoctoral fellow Dawei Lu has spent most of his research career working in circuit model quantum computing. The thought of transferring that knowledge to topological quantum computing intrigued him.

In a circuit model, you prepare your system for some kind of input, then build gates through which that input flows. You encode information at the start and decode it at the end. In a topological system, you have to design the gates as how the anyons braid with one another as they move through time. Then, you need to manipulate the anyons to move around, following the pre-designed paths.

In both instances, Lu said, you have to initialize the system, and then decode the information at the end. In broad terms, that makes them equivalent, he said.

“Before this work, to my best knowledge, I think people always considered these two approaches independently,” said Lu, who will begin a professorship at the Southern University of Science and Technology in Shenzhen, China, this August. “People know they are equivalent, but they seldom consider the connection between the two. This work shows that, even when you use a circuit-based model, you can still simulate the topological stuff.”

The experiment didn’t aim to perform topological computation. Rather, the researchers sought to simulate different topological systems, and then to identify one from the other. Lu describes the experiment in terms of meeting identical triplets: there’s no obvious way to tell them apart, so you have to dig deeper to find a more nuanced difference.

To do this, Lu and graduate student Keren Li prepared three systems, each made of room-temperature trichloroethylene molecules that simulated specific kinds of topological order (one of which was the non-Abelian Fibonacci anyon system).

IQC PhD student Keren Li and postdoctoral fellow Dawei Lu in the lab where they tested the TQC theory.[/caption]

Using a nuclear magnetic resonance quantum simulator, housed in the University of Waterloo chemistry building, they then rotated the molecules to mimic how anyons would move around the most basic lattice you can construct on a doughnut-shaped torus.

After each rotation, or “modular transformation,” they measured the new ground state. By working out the inner mathematical products that led from one ground state to the next, they were able to construct the specific matrix that determined each system’s inner workings.

The results showed distinct differences between the systems that, at the outset, had appeared identical. And the experimental results almost perfectly matched the theoretical predictions. They had found the fingerprints.

Forging a new path for discovery

This is just the first step in a longer research collaboration. The three circuit-model qubits being manipulated in this experiment simulate just one TQC qubit. To actually simulate excitations in a topological system, Wan predicts they’ll need at least six qubits. Braiding will require even more again.

What this experiment does do, however, is show that, while topological quantum computation might not be achievable tomorrow, simulations can help map out a path for exploration.

“I think it offers a new route to realize quantum computation,” Wan said. “If you have only 20 qubits and you want to realize a quantum circuit model, then probably only one or two qubits are used for real quantum computation. The others are for error correction. But to do this [TQC simulation], most of the qubits can be used for computation. That’s the best part of this proposal.”

Seeking out collaborations like this is not a typical trajectory for a theorist like Wan, but he sees it as something of a responsibility. If he doesn’t push to make his theories applicable, he reasons, their potential impact could be delayed – or missed entirely.

Having started his career in the extremely abstract area of quantum gravity, he plans to now divide his research time in two, devoting half to theory and the other half to experimental collaborations.

“This abstract stuff, without application, without experiment, would mean nothing to the world,” he said. “I have to find some true physical applications.”