Call it a twist ending.

Since 2009, experts have been debating whether a tensor network called MERA could be the key to a better understanding of the holographic principle – the deep mystery that proposes connections between field theory and quantum gravity.

The question was: if MERA is a key, then how does the key fit? Phrased more technically, experts were debating the exact relationship between MERA and holography. Two camps developed two theories about that relationship – call them option A and option B. Now, a pair of Perimeter researchers has provided a definitive answer.

They picked option C.

Introducing MERA

Faculty member Guifre Vidal and postdoctoral researcher Ash Milsted are experts in MERA. Milsted is the first postdoc hired under the Tensor Networks Initiative, Perimeter’s five-year push into tensor networks. Now finishing his postdoctoral fellowship – and soon off to a second postdoc at Caltech – he’s worked on developing MERA and other tensor network formalisms and applying them to a large number of other research areas, particularly in high energy physics.

Vidal is the researcher who originally developed MERA, which stands for ‘multi-scale entanglement renormalization ansatz.’ MERA is a mathematical structure that brings the tools of the renormalization group to bear on entangled quantum systems.

To take that piece by piece: the renormalization group is a set of tools that allow you to go from high energy physics to low energy physics. “It’s one of the most important ideas of the twentieth century,” says Vidal. “It connects physics at one length scale to another length scale.”

Entanglement, meanwhile, is a uniquely quantum kind of correlation, in which two or more particles are intertwined, such that the quantum state of each particle cannot be described independently of the state of the other.

Entangled particles don’t just come in pairs: they come in systems, and some of the systems are big. These systems can’t be understood piece by piece: that would be like trying to understand an image by understanding each pixel, or trying to understand a sentence by understanding each letter. As with the image or the sentence, in certain highly entangled systems, all the interesting stuff is in the relationships between the parts.

Since Vidal introduced it in 2005, MERA has become the tool of choice for understanding not just highly entangled quantum matter but a variety of other complex systems. It’s used in machine learning, in describing exotic states of matter, and in field theory, just to name a few.

The tool’s power goes back to a key insight Vidal had when developing it: he added an extra dimension to an older framework and used it to encode entanglement at different length scales. This was the origin of MERA, with its M for ‘multi-scale.’

MERA meets holography

Vidal’s seminal papers on MERA were published in 2007 and 2008. In 2009, another physicist named Brian Swingle noticed the parallels between the way MERA makes complex problems more tractable by adding a dimension and the way string theory does the same through its holographic principle.

The holographic principle says that certain kinds of field theories are exactly equivalent to certain kinds of gravitational theories in one more dimension. Also known as AdS/CFT, holography is central to the study of string theory and certain branches of field theory and gravity, and it has surprising uses far beyond them. The more Swingle looked, the deeper and more profound the parallels between MERA and holography seemed.

A community of research sprang up to build on Swingle’s initial insight. The researchers involved hoped that MERA would prove to be the formalism that could chop the complex mathematics of holography into manageable pieces, where calculations can actually be performed. In the parlance of the field: it was hoped that MERA would prove to be a lattice realization of AdS/CFT.

Lattice = progress

A realization, says Vidal, is simply a concrete version of something – “the way you might see ‘happiness’ realized in a little kid playing.” A lattice realization is one that can be considered piece by piece, rather than needing to be tackled as a whole.

Finding a lattice realization of holography would be a huge step forward. Milsted explains: “It would be a computational framework to answer questions. Sometimes just thinking is not good enough. You need to also be able to calculate things. If you can turn these abstract ideas in AdS/CFT into some lattice simulation, then you can put actual numbers in your computer. Then you are forced to be really concrete – forced to work out exactly what you mean.”

“Once you have chopped AdS/CFT into pieces, you can look at the pieces and ask questions,” says Vidal. “AdS/CFT can be very abstract. There are no experiments – the energies are too high to experiment with – so it’s possible to get lost in a castle of conjectures. It’s very useful to be able to chop the big construction into small pieces to understand what you meant in the first place.”

Option A, Option B – none of the above

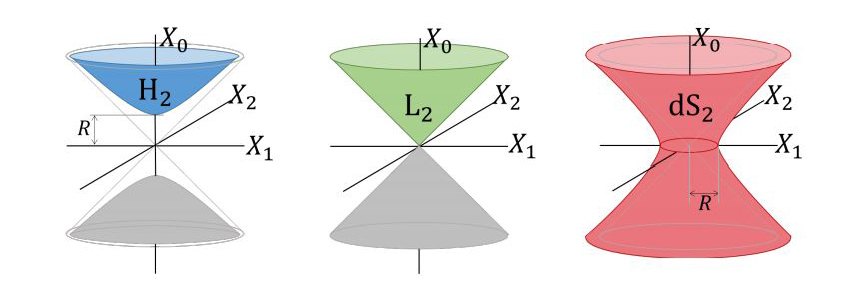
With the motivation strong and the connection widely sensed, the open question seemed to be exactly how to make sense of MERA in the holographic setting – whether it represented a theory of gravity in hyperbolic space as proposed by Swingle, or a field theory in de Sitter spacetime as proposed by Cedric Beny: whether it was option A or option B.

Now, after 10 years of debate, Vidal and Milsted finally have a definitive answer. MERA corresponds to neither hyperbolic space nor de Sitter spacetimes, but to a light cone geometry. It’s option C.

As a bonus – or a consolation prize – Milsted and Vidal developed two variants of MERA, one for use in in option A and one for use in option B. “So everyone can be happy,” says Vidal.

One might naively expect this breakthrough to rapidly advance the progress of holography. “Yes, I’d expect that too,” says Vidal. “But it might not be quite that simple.”

Though this new work finally establishes how to interpret MERA geometrically from a CFT perspective, researchers are still uncertain about how this new interpretation relates to the geometry of AdS/CFT. It looks as if the relationship is not as straightforward as previously believed.

“One of the implications of our work is that these tensor networks are not necessarily related to AdS/CFT. We’re not saying they’re not, we’re saying ‘where is this relationship?’” says Milsted.

“What our results show is what the exact relationships between the MERA and the CFT side of the story are,” adds Vidal. “It’s for the experts in AdS/CFT to find the gravitational side of the story.”

Clearly, the long and twisting tale of MERA and holography is not over yet.